Monoidal functor

In category theory, monoidal functors are functors between monoidal categories which preserve the monoidal structure. More specifically, a monoidal functor between two monoidal categories consists of a functor between the categories, along with two coherence maps—a natural transformation and a morphism that preserve monoidal multiplication and unit, respectively. Mathematicians require these coherence maps to satisfy additional properties depending on how strictly they want to preserve the monoidal structure; each of these properties gives rise to a slightly different definition of monoidal functors

• The coherence maps of lax monoidal functors satisfy no additional properties; they are not necessarily invertible.
• The coherence maps of strong monoidal functors are invertible.
• The coherence maps of strict monoidal functors are identity maps.

Although we distinguish between these different definitions here, authors may call any one of these simply monoidal functors.

Definition

Let ${\displaystyle ({\mathcal {C}},\otimes ,I_{\mathcal {C}})}$ and ${\displaystyle ({\mathcal {D}},\bullet ,I_{\mathcal {D}})}$ be monoidal categories. A lax monoidal functor from ${\displaystyle {\mathcal {C}}}$ to ${\displaystyle {\mathcal {D}}}$ (which may also just be called a monoidal functor) consists of a functor ${\displaystyle F:{\mathcal {C}}\to {\mathcal {D}}}$ together with a natural transformation

${\displaystyle \phi _{A,B}:FA\bullet FB\to F(A\otimes B)}$

between functors ${\displaystyle {\mathcal {C}}\times {\mathcal {C}}\to {\mathcal {D}}}$ and a morphism

${\displaystyle \phi :I_{\mathcal {D}}\to FI_{\mathcal {C}}}$,

called the coherence maps or structure morphisms, which are such that for every three objects ${\displaystyle A}$, ${\displaystyle B}$ and ${\displaystyle C}$ of ${\displaystyle {\mathcal {C}}}$ the diagrams

,

   and   

commute in the category ${\displaystyle {\mathcal {D}}}$. Above, the various natural transformations denoted using ${\displaystyle \alpha ,\rho ,\lambda }$ are parts of the monoidal structure on ${\displaystyle {\mathcal {C}}}$ and ${\displaystyle {\mathcal {D}}}$.

Variants

• The dual of a monoidal functor is a comonoidal functor; it is a monoidal functor whose coherence maps are reversed. Comonoidal functors may also be called opmonoidal, colax monoidal, or oplax monoidal functors.
• A strong monoidal functor is a monoidal functor whose coherence maps ${\displaystyle \phi _{A,B},\phi }$ are invertible.
• A strict monoidal functor is a monoidal functor whose coherence maps are identities.
• A braided monoidal functor is a monoidal functor between braided monoidal categories (with braidings denoted ${\displaystyle \gamma }$) such that the following diagram commutes for every pair of objects A, B in ${\displaystyle {\mathcal {C}}}$ :

• A symmetric monoidal functor is a braided monoidal functor whose domain and codomain are symmetric monoidal categories.

Examples

• The underlying functor ${\displaystyle U\colon (\mathbf {Ab} ,\otimes _{\mathbf {Z} },\mathbf {Z} )\rightarrow (\mathbf {Set} ,\times ,\{\ast \})}$ from the category of abelian groups to the category of sets. In this case, the map ${\displaystyle \phi _{A,B}\colon U(A)\times U(B)\to U(A\otimes B)}$ sends (a, b) to ${\displaystyle a\otimes b}$; the map ${\displaystyle \phi \colon \{*\}\to \mathbb {Z} }$ sends ${\displaystyle \ast }$ to 1.
• If ${\displaystyle R}$ is a (commutative) ring, then the free functor ${\displaystyle {\mathsf {Set}},\to R{\mathsf {-mod}}}$ extends to a strongly monoidal functor ${\displaystyle ({\mathsf {Set}},\sqcup ,\emptyset )\to (R{\mathsf {-mod}},\oplus ,0)}$ (and also ${\displaystyle ({\mathsf {Set}},\times ,\{\ast \})\to (R{\mathsf {-mod}},\otimes ,R)}$ if ${\displaystyle R}$ is commutative).
• If ${\displaystyle R\to S}$ is a homomorphism of commutative rings, then the restriction functor ${\displaystyle (S{\mathsf {-mod}},\otimes _{S},S)\to (R{\mathsf {-mod}},\otimes _{R},R)}$ is monoidal and the induction functor ${\displaystyle (R{\mathsf {-mod}},\otimes _{R},R)\to (S{\mathsf {-mod}},\otimes _{S},S)}$ is strongly monoidal.
• An important example of a symmetric monoidal functor is the mathematical model of topological quantum field theory, which has been recently developed. Let ${\displaystyle \mathbf {Bord} _{\langle n-1,n\rangle }}$ be the category of cobordisms of n-1,n-dimensional manifolds with tensor product given by disjoint union, and unit the empty manifold. A topological quantum field theory in dimension n is a symmetric monoidal functor ${\displaystyle F\colon (\mathbf {Bord} _{\langle n-1,n\rangle },\sqcup ,\emptyset )\rightarrow (\mathbf {kVect} ,\otimes _{k},k).}$
• The homology functor is monoidal as ${\displaystyle (Ch(R{\mathsf {-mod}}),\otimes ,R[0])\to (grR{\mathsf {-mod}},\otimes ,R[0])}$ via the map ${\displaystyle H_{\ast }(C_{1})\otimes H_{\ast }(C_{2})\to H_{\ast }(C_{1}\otimes C_{2}),[x_{1}]\otimes [x_{2}]\mapsto [x_{1}\otimes x_{2}]}$.

Alternate notions

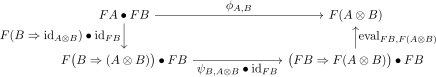
If ${\displaystyle ({\mathcal {C}},\otimes ,I_{\mathcal {C}})}$ and ${\displaystyle ({\mathcal {D}},\bullet ,I_{\mathcal {D}})}$ are closed monoidal categories with internal hom-functors ${\displaystyle \Rightarrow _{\mathcal {C}},\Rightarrow _{\mathcal {D}}}$ (we drop the subscripts for readability), there is an alternative formulation

ψ_AB : F(A ⇒ B) → FA ⇒ FB

of φ_AB commonly used in functional programming. The relation between ψ_AB and φ_AB is illustrated in the following commutative diagrams:

Properties

• If ${\displaystyle (M,\mu ,\epsilon )}$ is a monoid object in ${\displaystyle C}$, then ${\displaystyle (FM,F\mu \circ \phi _{M,M},F\epsilon \circ \phi )}$ is a monoid object in ${\displaystyle D}$.

Monoidal functors and adjunctions

Suppose that a functor ${\displaystyle F:{\mathcal {C}}\to {\mathcal {D}}}$ is left adjoint to a monoidal ${\displaystyle (G,n):({\mathcal {D}},\bullet ,I_{\mathcal {D}})\to ({\mathcal {C}},\otimes ,I_{\mathcal {C}})}$. Then ${\displaystyle F}$ has a comonoidal structure ${\displaystyle (F,m)}$ induced by ${\displaystyle (G,n)}$, defined by

${\displaystyle m_{A,B}=\varepsilon _{FA\bullet FB}\circ Fn_{FA,FB}\circ F(\eta _{A}\otimes \eta _{B}):F(A\otimes B)\to FA\bullet FB}$

and

${\displaystyle m=\varepsilon _{I_{\mathcal {D}}}\circ Fn:FI_{\mathcal {C}}\to I_{\mathcal {D}}}$.

If the induced structure on ${\displaystyle F}$ is strong, then the unit and counit of the adjunction are monoidal natural transformations, and the adjunction is said to be a monoidal adjunction; conversely, the left adjoint of a monoidal adjunction is always a strong monoidal functor.

Similarly, a right adjoint to a comonoidal functor is monoidal, and the right adjoint of a comonoidal adjunction is a strong monoidal functor.

See also

• Monoidal natural transformation

References

• Kelly, G. Max (1974). "Doctrinal adjunction". Category Seminar. Lecture Notes in Mathematics. Vol. 420. Springer. pp. 257–280. doi:10.1007/BFb0063105. ISBN 978-3-540-37270-7.